Abstract:

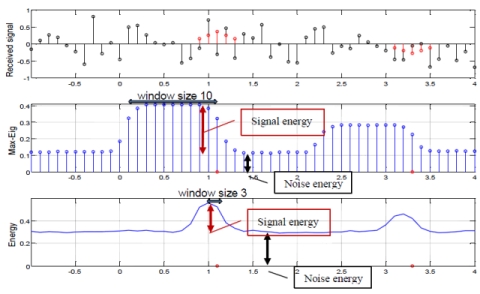
Due to the fine delay resolution in ultra-wideband (UWB) wireless propagation channels, a large number of multipath components (MPC) can be resolved; and the first arriving MPC might not be the strongest one. This makes time-of-arrival (ToA) estimation, which essentially depends on determining the arrival time of the first MPC, highly challenging. In this paper, we consider non-coherent ToA estimation given a number of measurement trials, at moderate sampling rate and in the absence of knowledge of pulse shape. The proposed ToA estimation is based on detecting the presence of a signal in a moving time delay window, by using the largest eigenvalue of the sample covariance matrix of the signal in the window as the test statistic. We show that energy detection can be viewed as a special case of the eigenvalue detection. Maxeigenvalue detection (MED) generally has superior performance, due to the following reasons: 1) MED collects less noise, namely only the noise contained in the signal space, and 2) if multiple channel taps fall into the time window, the MED detector can collect energy from all of them. Simulation results confirm that MED outperforms the energy detection in IEEE 802.15.3a and 802.15.4a channels. Finally, the selection of the threshold of the MED is studied both by simulations and by random matrix theory.